- Electromagnetics I
- Ch 5
- Loc 5.19
Charge and Electric Field for a Perfectly Conducting Region
In this section, we consider the behavior of charge and the electric field in the vicinity of a perfect electrical conductor (PEC).
First, note that the electric field – both the electric field intensity E and electric flux density D – throughout a PEC region is zero. This is because the electrical potential throughout a PEC region must be constant. (This idea is explored further in Section 6.3). Recall that the electric field is proportional to the spatial rate of change of electrical potential (i.e.,

; Section 5.14). Thus, the electric field must be zero throughout a PEC region.
Second, the electric field is oriented directly away from (i.e., perpendicular to) the PEC surface, and the magnitude of

is equal to the surface charge density

(C/m

) (Section 5.18)
Now we address the question of charge distribution; i.e., the location and density of charge. Consider the scenario shown in Figure 5.19.1.
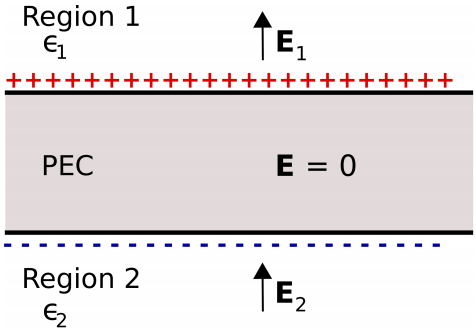
Figure 5.19.1: An infinite flat slab of PEC in the presence of an applied electric field. Image used with permission (CC BY SA 4.0; K. Kikkeri).
Here, a flat slab of PEC material is embedded in dielectric material.1 The thickness of the slab is finite, whereas the length and width of the slab is infinite. The region above the slab is defined as Region 1 and has permittivity

. The region below the slab is defined as Region 2 and has permittivity

. Electric fields

and

are present in Regions 1 and 2, respectively, as shown in Figure 5.19.1. To begin, let us assume that these fields are the result of some external stimulus that results in the direction of these fields being generally upward, as shown in Figure 5.19.1.
Now, what do we know about

and

? First, both fields must satisfy the relevant boundary conditions. That is, the component of

that is tangent to the upper PEC surface is zero, so that

is directed entirely in a direction perpendicular to the surface. Similarly, the component of

that is tangent to the lower PEC surface is zero, so that

is directed entirely in a direction perpendicular to the surface. At this point we have not determined the magnitudes or signs of

and

; we have established only that there are no non-zero components tangential to (i.e., parallel to) the PEC surfaces.
Next, recall that the electric field must be zero within the slab. This means that there must be zero net charge within the slab, since any other distribution of charge will result in a non-zero electric field, and subsequently a potential difference between locations within the slab. Therefore:
There can be no static charge within a PEC.
It follows that
Charge associated with a PEC lies entirely on the surface.
Outside the slab, the boundary conditions on

and

in the dielectric regions require these fields to be non-zero when the surface charge density on the PEC is non-zero. The surface charge supports the discontinuity in the normal component of the electric fields. Specifically,

and

have the same magnitude ∣∣ρs∣∣|ρs| because the surface charge densities on both sides of the slab have equal magnitude. However, the electric field intensity

, whereas

; i.e., these are different. That is, the electric field intensities are unequal unless the permittivities in each dielectric region are equal.
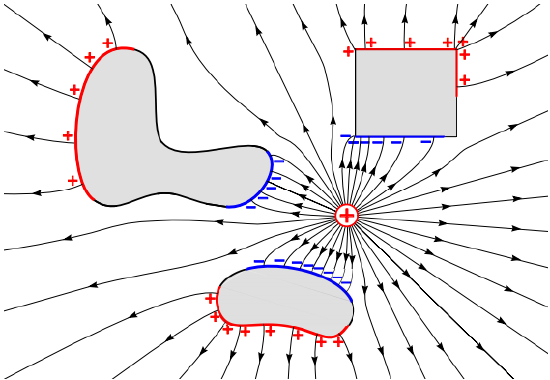
Figure 5.19.2: Electric field lines due to a point charge in the vicinity of PEC regions (shaded) of various shapes. Image used (by C. Burks).
Finally, let us consider the structure of the electric field in more general cases. Figure 5.19.2 shows field lines in a homogeneous dielectric material in which a point charge and PEC regions of various shapes are embedded. Note that electric field lines now bend in the dielectric so as to satisfy the requirement that the tangential component of the electric field be zero on PEC surfaces. Also note that the charge distribution arranges itself on the PEC surfaces so as to maintain zero electric field and constant potential within the cube.
Footnotes
Ellingson, Steven W. (2018) Electromagnetics, Vol. 1. Blacksburg, VA: VT Publishing. https://doi.org/10.21061/electromagnetics-vol-1 CC BY-SA 4.0
Explore CircuitBread

Get the latest tools and tutorials, fresh from the toaster.







