Stokes' Theorem

Stokes’ Theorem relates an integral over an open surface to an integral over the curve bounding that surface. This relationship has a number of applications in electromagnetic theory. Here is the theorem:

where

is the open surface bounded by the closed path

. The direction of the surface normal

is related to the direction of integration along

by the right-hand rule, illustrated in Figure 4.9.1. In this case, the right-hand rule states that the correct normal is the one that points through the surface in the same direction as the fingers of the right hand when the thumb of your right hand is aligned along

in the direction of integration.

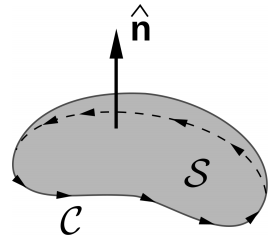
Figure 4.9.1: The relative orientations of the direction of integration C and surface normal nˆ in Stokes’ Theorem. Image used with permission (CC BY SA 3.0; Cronholm144).

About Stokes Theorem

Stokes’ Theorem is a purely mathematical result and not a principle of electromagnetics per se. The relevance of the theorem to electromagnetic theory is primarily as a tool in the associated mathematical analysis. Usually the theorem is employed to transform a problem expressed in terms of an integration over a surface into an integration over a closed path or vice-versa. For more information on the theorem and its derivation, see “Additional Reading” at the end of this section.