Chapter 3 Review

Key Terms

electric dipole
system of two equal but opposite charges a fixed distance apart

electric dipole moment
quantity defined as

for all dipoles, where the vector points from the negative to positive charge

electric potential
potential energy per unit charge

electric potential difference
the change in potential energy of a charge

moved between two points, divided by the charge.

electric potential energy
potential energy stored in a system of charged objects due to the charges

electron-volt
energy given to a fundamental charge accelerated through a potential difference of one volt

electrostatic precipitators
filters that apply charges to particles in the air, then attract those charges to a filter, removing them from the airstream

equipotential line
two-dimensional representation of an equipotential surface

equipotential surface
surface (usually in three dimensions) on which all points are at the same potential

grounding
process of attaching a conductor to the earth to ensure that there is no potential difference between it and Earth

ink jet printer
small ink droplets sprayed with an electric charge are controlled by electrostatic plates to create images on paper

photoconductor
substance that is an insulator until it is exposed to light, when it becomes a conductor

Van de Graaff generator
machine that produces a large amount of excess charge, used for experiments with high voltage

voltage
change in potential energy of a charge moved from one point to another, divided by the charge; units of potential difference are joules per coulomb, known as volt

xerography
dry copying process based on electrostatics

Key Equations

Potential energy of a two-charge system
Work done to assemble a system of charges
Potential difference	or
Electric potential
Potential difference between two points
Electric potential of a point charge
Electric potential of a system of point charges
Electric dipole moment
Electric potential due to a dipole
Electric potential of a continuous charge distribution
Electric field components
Del operator in Cartesian coordinates
Electric field as gradient of potential
Del operator in cylindrical coordinates
Del operator in spherical coordinates

Summary

3.1 Electric Potential Energy

• The work done to move a charge from point to in an electric field is path independent, and the work around a closed path is zero. Therefore, the electric field and electric force are conservative.
• We can define an electric potential energy, which between point charges is , with the zero reference taken to be at infinity.
• The superposition principle holds for electric potential energy; the potential energy of a system of multiple charges is the sum of the potential energies of the individual pairs.

3.2 Electric Potential and Potential Difference

• Electric potential is potential energy per unit charge.
• The potential difference between points and , , that is, the change in potential of a charge moved from to , is equal to the change in potential energy divided by the charge.
• Potential difference is commonly called voltage, represented by the symbol :
  
  
  
• An electron-volt is the energy given to a fundamental charge accelerated through a potential difference of . In equation form,
  
  

3.3 Calculations of Electric Potential

• Electric potential is a scalar whereas electric field is a vector.
• Addition of voltages as numbers gives the voltage due to a combination of point charges, allowing us to use the principle of superposition: .
• An electric dipole consists of two equal and opposite charges a fixed distance apart, with a dipole moment .
• Continuous charge distributions may be calculated with .

3.4 Determining Field from Potential

• Just as we may integrate over the electric field to calculate the potential, we may take the derivative of the potential to calculate the electric field.
• This may be done for individual components of the electric field, or we may calculate the entire electric field vector with the gradient operator.

3.5 Equipotential Surfaces and Conductors

• An equipotential surface is the collection of points in space that are all at the same potential. Equipotential lines are the two-dimensional representation of equipotential surfaces.
• Equipotential surfaces are always perpendicular to electric field lines.
• Conductors in static equilibrium are equipotential surfaces.
• Topographic maps may be thought of as showing gravitational equipotential lines.

3.6 Applications of Electrostatics

• Electrostatics is the study of electric fields in static equilibrium.
• In addition to research using equipment such as a Van de Graaff generator, many practical applications of electrostatics exist, including photocopiers, laser printers, ink jet printers, and electrostatic air filters.

Answers to Check Your Understanding

3.1.

3.2. It has kinetic energy of

at point

and potential energy of

which means that as

approaches infinity, its kinetic energy totals three times the kinetic energy at

since all of the potential energy gets converted to kinetic.

3.3. positive, negative, and these quantities are the same as the work you would need to do to bring the charges in from infinity

3.4.

3.5.

electrons

3.6. It would be going in the opposite direction, with no effect on the calculations as presented.

3.7. Given a fixed maximum electric field strength, the potential at which a strike occurs increases with increasing height above the ground. Hence, each electron will carry more energy. Determining if there is an effect on the total number of electrons lies in the future.

3.8.

recall that the electric field inside a conductor is zero. Hence, any path from a point on the surface to any point in the interior will have an integrand of zero when calculating the change in potential, and thus the potential in the interior of the sphere is identical to that on the surface.

3.9. The x-axis the potential is zero, due to the equal and opposite charges the same distance from it. On the

-axis, we may superimpose the two potentials; we will find that for

again the potential goes to zero due to cancellation.

3.10. It will be zero, as at all points on the axis, there are equal and opposite charges equidistant from the point of interest. Note that this distribution will, in fact, have a dipole moment.

3.11. Any, but cylindrical is closest to the symmetry of a dipole.

3.12. infinite cylinders of constant radius, with the line charge as the axis

Conceptual Questions

3.1 Electric Potential Energy

1. Would electric potential energy be meaningful if the electric field were not conservative?

2. Why do we need to be careful about work done on the system versus work done by the system in calculations?

3. Does the order in which we assemble a system of point charges affect the total work done?

3.2 Electric Potential and Potential Difference

4. Discuss how potential difference and electric field strength are related. Give an example.

5. What is the strength of the electric field in a region where the electric potential is constant?

6. If a proton is released from rest in an electric field, will it move in the direction of increasing or decreasing potential? Also answer this question for an electron and a neutron. Explain why.

7. Voltage is the common word for potential difference. Which term is more descriptive, voltage or potential difference?

8. If the voltage between two points is zero, can a test charge be moved between them with zero net work being done? Can this necessarily be done without exerting a force? Explain.

9. What is the relationship between voltage and energy? More precisely, what is the relationship between potential difference and electric potential energy?

10. Voltages are always measured between two points. Why?

11. How are units of volts and electron-volts related? How do they differ?

12. Can a particle move in a direction of increasing electric potential, yet have its electric potential energy decrease? Explain.

3.3 Calculations of Electric Potential

13. Compare the electric dipole moments of charges

separated by a distance

and charges

separated by a distance

.

14. Would Gauss’s law be helpful for determining the electric field of a dipole? Why?

15. In what region of space is the potential due to a uniformly charged sphere the same as that of a point charge? In what region does it differ from that of a point charge?

16. Can the potential of a nonuniformly charged sphere be the same as that of a point charge? Explain.

3.4 Determining Field from Potential

17. If the electric field is zero throughout a region, must the electric potential also be zero in that region?

18. Explain why knowledge of

is not sufficient to determine

What about the other way around?

3.5 Equipotential Surfaces and Conductors

19. If two points are at the same potential, are there any electric field lines connecting them?

20. Suppose you have a map of equipotential surfaces spaced

apart. What do the distances between the surfaces in a particular region tell you about the strength of the

in that region?

21. Is the electric potential necessarily constant over the surface of a conductor?

22. Under electrostatic conditions, the excess charge on a conductor resides on its surface. Does this mean that all of the conduction electrons in a conductor are on the surface?

23. Can a positively charged conductor be at a negative potential? Explain.

24. Can equipotential surfaces intersect?

3.6 Applications of Electrostatics

25. Why are the metal support rods for satellite network dishes generally grounded?

26. (a) Why are fish reasonably safe in an electrical storm? (b) Why are swimmers nonetheless ordered to get out of the water in the same circumstance?

27. What are the similarities and differences between the processes in a photocopier and an electrostatic precipitator?

28. About what magnitude of potential is used to charge the drum of a photocopy machine? A web search for “xerography” may be of use.

Problems

3.1 Electric Potential Energy

29. Consider a charge

fixed at a site with another charge

(charge

mass

) moving in the neighboring space. (a) Evaluate the potential energy of

when it is

from

(b) If

starts from rest from a point

from

what will be its speed when it is

from

? (Note:

is held fixed in its place.)

30. Two charges

and

are placed symmetrically along the

-axis at

Consider a charge

and mass

moving along the

-axis. If

starts from rest at

what is its speed when it reaches

?

31. To form a hydrogen atom, a proton is fixed at a point and an electron is brought from far away to a distance of

the average distance between proton and electron in a hydrogen atom. How much work is done?

32. (a) What is the average power output of a heart defibrillator that dissipates

of energy in

? (b) Considering the high-power output, why doesn’t the defibrillator produce serious burns?

3.2 Electric Potential and Potential Difference

33. Find the ratio of speeds of an electron and a negative hydrogen ion (one having an extra electron) accelerated through the same voltage, assuming non-relativistic final speeds. Take the mass of the hydrogen ion to be

34. An evacuated tube uses an accelerating voltage of

to accelerate electrons to hit a copper plate and produce X-rays. Non-relativistically, what would be the maximum speed of these electrons?

35. Show that units of

and

for electric field strength are indeed equivalent.

36. What is the strength of the electric field between two parallel conducting plates separated by

and having a potential difference (voltage) between them of

?

37. The electric field strength between two parallel conducting plates separated by

is

(a) What is the potential difference between the plates? (b) The plate with the lowest potential is taken to be zero volts. What is the potential

from that plate and

from the other?

38. The voltage across a membrane forming a cell wall is

and the membrane is

thick. What is the electric field strength? (The value is surprisingly large, but correct.) You may assume a uniform electric field.

39. Two parallel conducting plates are separated by

, and one of them is taken to be at zero volts. (a) What is the electric field strength between them, if the potential

from the zero volt plate (and

from the other) is

? (b) What is the voltage between the plates?

40. Find the maximum potential difference between two parallel conducting plates separated by

of air, given the maximum sustainable electric field strength in air to be

41. An electron is to be accelerated in a uniform electric field having a strength of

(a) What energy in

is given to the electron if it is accelerated through

? (b) Over what distance would it have to be accelerated to increase its energy by

?

42. Use the definition of potential difference in terms of electric field to deduce the formula for potential difference between

and

for a point charge located at the origin. Here

is the spherical radial coordinate.

43. The electric field in a region is pointed away from the

-axis and the magnitude depends upon the distance

from the axis. The magnitude of the electric field is given as

where

is a constant. Find the potential difference between points

and

explicitly stating the path over which you conduct the integration for the line integral.

44. Singly charged gas ions are accelerated from rest through a voltage of

. At what temperature will the average kinetic energy of gas molecules be the same as that given these ions?

3.3 Calculations of Electric Potential

45. A

-diameter plastic sphere, used in a static electricity demonstration, has a uniformly distributed

charge on its surface. What is the potential near its surface?

46. How far from a

point charge is the potential

? At what distance is it

?

47. If the potential due to a point charge is

at a distance of

what are the sign and magnitude of the charge?

48. In nuclear fission, a nucleus splits roughly in half. (a) What is the potential

from a fragment that has

protons in it? (b) What is the potential energy in

of a similarly charged fragment at this distance?

49. A research Van de Graaff generator has a

-diameter metal sphere with a charge of

on it. (a) What is the potential near its surface? (b) At what distance from its center is the potential

? (c) An oxygen atom with three missing electrons is released near the Van de Graaff generator. What is its energy in

when the atom is at the distance found in part b?

50. An electrostatic paint sprayer has a

-diameter metal sphere at a potential of

that repels paint droplets onto a grounded object. (a) What charge is on the sphere? (b) What charge must a

drop of paint have to arrive at the object with a speed of

?

51. (a) What is the potential between two points situated

and

from a

point charge? (b) To what location should the point at

be moved to increase this potential difference by a factor of two?

52. Find the potential at points

and

in the diagram due to the two given charges.

53. Two charges

and

are separated by

on the

-axis symmetrically about origin, with the positive one uppermost. Two space points of interest

and

are located

and

from origin at an angle

with respect to the

-axis. Evaluate electric potentials at

and

in two ways: (a) Using the exact formula for point charges, and (b) using the approximate dipole potential formula.

54. (a) Plot the potential of a uniformly charged

rod with

charge as a function of the perpendicular distance from the centre. Draw your graph from

to

(b) On the same graph, plot the potential of a point charge with a

charge at the origin. (c) Which potential is stronger near the rod? (d) What happens to the difference as the distance increases? Interpret your result.

3.4 Determining Field from Potential

55. Throughout a region, equipotential surfaces are given by

The surfaces are equally spaced with

for

for

for

What is the electric field in this region?

56. In a particular region, the electric potential is given by

What is the electric field in this region?

57. Calculate the electric field of an infinite line charge, throughout space.

3.5 Equipotential Surfaces and Conductors

58. Two very large metal plates are placed

apart, with a potential difference of

between them. Consider one plate to be at

and the other at

(a) Sketch the equipotential surfaces for

and

(b) Next sketch in some electric field lines, and confirm that they are perpendicular to the equipotential lines.

59. A very large sheet of insulating material has had an excess of electrons placed on it to a surface charge density of

(a) As the distance from the sheet increases, does the potential increase or decrease? Can you explain why without any calculations? Does the location of your reference point matter? (b) What is the shape of the equipotential surfaces? (c) What is the spacing between surfaces that differ by

?

60. A metallic sphere of radius

is charged with

charge, which spreads on the surface of the sphere uniformly. The metallic sphere stands on an insulated stand and is surrounded by a larger metallic spherical shell, of inner radius

and outer radius

Now, a charge of

is placed on the inside of the spherical shell, which spreads out uniformly on the inside surface of the shell. If potential is zero at infinity, what is the potential of (a) the spherical shell, (b) the sphere, (c) the space between the two, (d) inside the sphere, and (e) outside the shell?

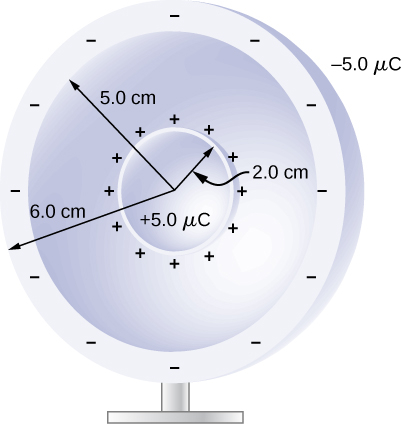
61. Two large charged plates of charge density

face each other at a separation of

(a) Find the electric potential everywhere. (b) An electron is released from rest at the negative plate; with what speed will it strike the positive plate?

62. A long cylinder of aluminum of radius

meters is charged so that it has a uniform charge per unit length on its surface of

(a) Find the electric field inside and outside the cylinder. (b) Find the electric potential inside and outside the cylinder. (c) Plot electric field and electric potential as a function of distance from the centre of the rod.

63. Two parallel plates

on a side are given equal and opposite charges of magnitude

The plates are

apart. What is the potential difference between the plates?

64. The surface charge density on a long straight metallic pipe is

What is the electric potential outside and inside the pipe? Assume the pipe has a diameter of

65. Concentric conducting spherical shells carry charges

and

respectively. The inner shell has negligible thickness. What is the potential difference between the shells?

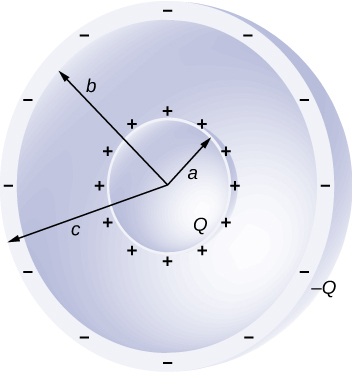
66. Shown below are two concentric spherical shells of negligible thicknesses and radii

and

The inner and outer shell carry net charges

and

respectively, where both

and

are positive. What is the electric potential in the regions (a)

(b)

and (c)

?

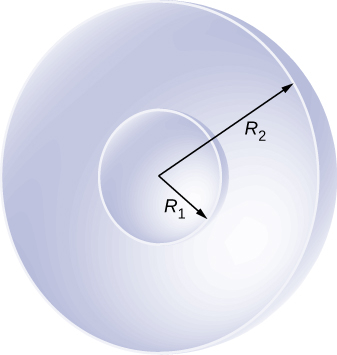
67. A solid cylindrical conductor of radius a is surrounded by a concentric cylindrical shell of inner radius

. The solid cylinder and the shell carry charges

and

respectively. Assuming that the length

of both conductors is much greater than

or

what is the potential difference between the two conductors?

3.6 Applications of Electrostatics

68. (a) What is the electric field

from the centre of the terminal of a Van de Graaff with a

charge, noting that the field is equivalent to that of a point charge at the centre of the terminal? (b) At this distance, what force does the field exert on a

charge on the Van de Graaff’s belt?

69. (a) What is the direction and magnitude of an electric field that supports the weight of a free electron near the surface of Earth? (b) Discuss what the small value for this field implies regarding the relative strength of the gravitational and electrostatic forces.

70. A simple and common technique for accelerating electrons is shown in Figure 3.7.1, where there is a uniform electric field between two plates. Electrons are released, usually from a hot filament, near the negative plate, and there is a small hole in the positive plate that allows the electrons to continue moving. (a) Calculate the acceleration of the electron if the field strength is

(b) Explain why the electron will not be pulled back to the positive plate once it moves through the hole.

(Figure 3.7.1)

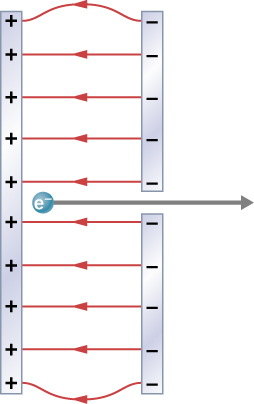
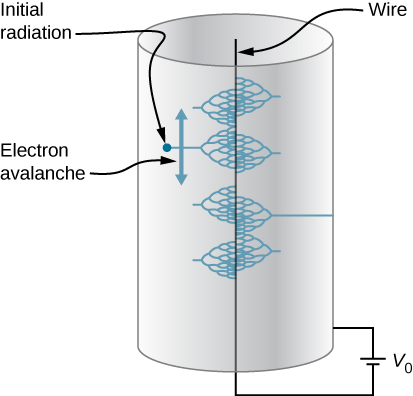
71. In a Geiger counter, a thin metallic wire at the center of a metallic tube is kept at a high voltage with respect to the metal tube. Ionizing radiation entering the tube knocks electrons off gas molecules or sides of the tube that then accelerate towards the center wire, knocking off even more electrons. This process eventually leads to an avalanche that is detectable as a current. A particular Geiger counter has a tube of radius

and the inner wire of radius

is at a potential of

volts with respect to the outer metal tube. Consider a point

at a distance

from the centre wire and far away from the ends. (a) Find a formula for the electric field at a point

inside using the infinite wire approximation. (b) Find a formula for the electric potential at a point

inside. (c) Use

and find the value of the electric field at a point

from the centre.

72. The practical limit to an electric field in air is about

Above this strength, sparking takes place because air begins to ionize. (a) At this electric field strength, how far would a proton travel before hitting the speed of light (ignore relativistic effects)? (b) Is it practical to leave air in particle accelerators?

73. To form a helium atom, an alpha particle that contains two protons and two neutrons is fixed at one location, and two electrons are brought in from far away, one at a time. The first electron is placed at

from the alpha particle and held there while the second electron is brought to

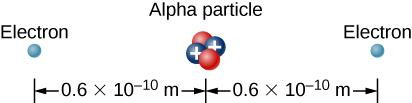
from the alpha particle on the other side from the first electron. See the final configuration below. (a) How much work is done in each step? (b) What is the electrostatic energy of the alpha particle and two electrons in the final configuration?

74. Find the electrostatic energy of eight equal charges

each fixed at the corners of a cube of side

75. The probability of fusion occurring is greatly enhanced when appropriate nuclei are brought close together, but mutual Coulomb repulsion must be overcome. This can be done using the kinetic energy of high-temperature gas ions or by accelerating the nuclei toward one another. (a) Calculate the potential energy of two singly charged nuclei separated by

(b) At what temperature will atoms of a gas have an average kinetic energy equal to this needed electrical potential energy?

76. A bare helium nucleus has two positive charges and a mass of

(a) Calculate its kinetic energy in joules at

of the speed of light. (b) What is this in electron-volts? (c) What voltage would be needed to obtain this energy?

77. An electron enters a region between two large parallel plates made of aluminum separated by a distance of

and kept at a potential difference of

The electron enters through a small hole in the negative plate and moves toward the positive plate. At the time the electron is near the negative plate, its speed is

Assume the electric field between the plates to be uniform, and find the speed of electron at (a)

(b)

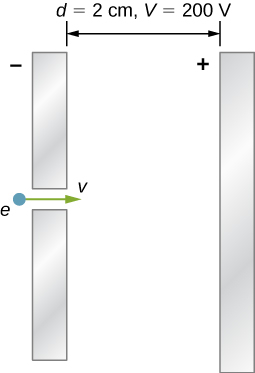
(c)

and (d)

from the negative plate, and (e) immediately before it hits the positive plate.

78. How far apart are two conducting plates that have an electric field strength of

between them, if their potential difference is

?

79. (a) Will the electric field strength between two parallel conducting plates exceed the breakdown strength of dry air, which is

if the plates are separated by

and a potential difference of

is applied? (b) How close together can the plates be with this applied voltage?80. Membrane walls of living cells have surprisingly large electric fields across them due to separation of ions. What is the voltage across an

-thick membrane if the electric field strength across it is

? You may assume a uniform electric field.

81. A double charged ion is accelerated to an energy of

by the electric field between two parallel conducting plates separated by

What is the electric field strength between the plates?

82. The temperature near the centre of the Sun is thought to be

million degrees Celsius

(or kelvin). Through what voltage must a singly charged ion be accelerated to have the same energy as the average kinetic energy of ions at this temperature?

83. A lightning bolt strikes a tree, moving

of charge through a potential difference of

(a) What energy was dissipated? (b) What mass of water could be raised from

to the boiling point and then boiled by this energy? (c) Discuss the damage that could be caused to the tree by the expansion of the boiling steam.

84. What is the potential

from a proton (the average distance between the proton and electron in a hydrogen atom)?

85. (a) A sphere has a surface uniformly charged with

. At what distance from its centre is the potential

? (b) What does your answer imply about the practical aspect of isolating such a large charge?86. What are the sign and magnitude of a point charge that produces a potential of

at a distance of

?

87. In one of the classic nuclear physics experiments at the beginning of the twentieth century, an alpha particle was accelerated toward a gold nucleus, and its path was substantially deflected by the Coulomb interaction. If the energy of the doubly charged alpha nucleus was

how close to the gold nucleus (

protons) could it come before being deflected?

Additional Problems

88. A

battery-operated bottle warmer heats

of glass,

of baby formula, and

of aluminum from

to

(a) How much charge is moved by the battery? (b) How many electrons per second flow if it takes

to warm the formula? (Hint: Assume that the specific heat of baby formula is about the same as the specific heat of water.)

89. A battery-operated car uses a

system. Find the charge the batteries must be able to move in order to accelerate the

car from rest to

make it climb a

high hill, and finally cause it to travel at a constant

while climbing with

force for an hour.

90. (a) Find the voltage near a

diameter metal sphere that has

of excess positive charge on it. (b) What is unreasonable about this result? (c) Which assumptions are responsible?

91. A uniformly charged ring of radius

is placed on a nonconducting table. It is found that

above the centre of the half-ring the potential is

with respect to zero potential at infinity. How much charge is in the half-ring?

92. A glass ring of radius

is painted with a charged paint such that the charge density around the ring varies continuously given by the following function of the polar angle

Find the potential at a point

above the centre.

93. A compact disk of radius

is sprayed with a charged paint so that the charge varies continually with radial distance

from the centre in the following manner:

Find the potential at a point

above the centre.

94. (a) What is the final speed of an electron accelerated from rest through a voltage of

by a negatively charged Van de Graff terminal? (b) What is unreasonable about this result? (c) Which assumptions are responsible?

95. A large metal plate is charged uniformly to a density of

How far apart are the equipotential surfaces that represent a potential difference of

?

96. Your friend gets really excited by the idea of making a lightning rod or maybe just a sparking toy by connecting two spheres as shown in Figure 3.5.10, and making

so small that the electric field is greater than the dielectric strength of air, just from the usual

electric field near the surface of the Earth. If

is

how small does

need to be, and does this seem practical?

97. (a) Find

limit of the potential of a finite uniformly charged rod and show that it coincides with that of a point charge formula. (b) Why would you expect this result?

98. A small spherical pith ball of radius

is painted with a silver paint and then

of charge is placed on it. The charged pith ball is put at the centre of a gold spherical shell of inner radius

and outer radius

(a) Find the electric potential of the gold shell with respect to zero potential at infinity. (b) How much charge should you put on the gold shell if you want to make its potential

?

99. Two parallel conducting plates, each of cross-sectional area

are

apart and uncharged. If

electrons are transferred from one plate to the other, (a) what is the potential difference between the plates? (b) What is the potential difference between the positive plate and a point

from it that is between the plates?

100. A point charge of

is placed at the centre of an uncharged spherical conducting shell of inner radius

and outer radius

Find the electric potential at (a)

(b)

(c)

101. Earth has a net charge that produces an electric field of approximately

downward at its surface. (a) What is the magnitude and sign of the excess charge, noting the electric field of a conducting sphere is equivalent to a point charge at its centre? (b) What acceleration will the field produce on a free electron near Earth’s surface? (c) What mass object with a single extra electron will have its weight supported by this field?

102. Point charges of

and

are placed

apart. (a) At what point along the line between them is the electric field zero? (b) What is the electric field halfway between them?

103. What can you say about two charges

and

if the electric field one-fourth of the way from

to

is zero?

104. Calculate the angular velocity

of an electron orbiting a proton in the hydrogen atom, given the radius of the orbit is

You may assume that the proton is stationary and the centripetal force is supplied by Coulomb attraction.

105. An electron has an initial velocity of

in a uniform

electric field. The field accelerates the electron in the direction opposite to its initial velocity. (a) What is the direction of the electric field? (b) How far does the electron travel before coming to rest? (c) How long does it take the electron to come to rest? (d) What is the electron’s velocity when it returns to its starting point?

Challenge Problems

106. Three

and three

ions are placed alternately and equally spaced around a circle of radius

. Find the electrostatic energy stored.

107. Look up (presumably online, or by dismantling an old device and making measurements) the magnitude of the potential deflection plates (and the space between them) in an ink jet printer. Then look up the speed with which the ink comes out the nozzle. Can you calculate the typical mass of an ink drop?

108. Use the electric field of a finite sphere with constant volume charge density to calculate the electric potential, throughout space. Then check your results by calculating the electric field from the potential.

109. Calculate the electric field of a dipole throughout space from the potential.

Candela Citations

CC licensed content, Specific attribution

• Download for free at http://cnx.org/contents/7a0f97...; Retrieved from: http://cnx.org/contents/7a0f97...; License: CC BY: Attribution