Inductance of a Straight Coil

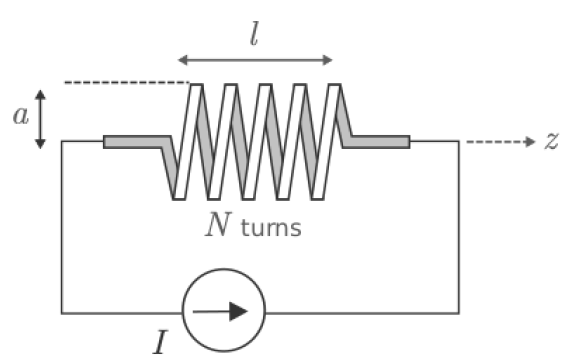
In this section, we determine the inductance of a straight coil, as shown in Figure 7.13.1. The coil is circular with radius

and length

and consists of

windings of wire wound with uniform winding density. Also, we assume the winding density

is large enough that magnetic field lines cannot enter or exit between windings but rather must traverse the entire length of the coil. Since the coil forms a cylinder, the problem is easiest to work in cylindrical coordinates with the axis of the coil aligned along the

axis.

Inductance

in this case is given by (Section 7.12)

where

is current and

is the magnetic flux associated with one winding of the coil. Magnetic flux in this case is given by

where

is the magnetic flux density (units of T = Wb/m²),

is the surface bounded by a single current loop, and

points in the direction determined by the right hand rule with respect to the direction of positive current flow.

First, let’s determine the magnetic field. The magnetic flux density deep inside the coil is (Section 7.6):

Is it reasonable to use this approximation here? Since inductance pertains to energy storage, the question is really what fraction of the energy is stored in a field that is well-described by this approximation, as opposed to energy stored in the “fringing field” close to the ends of the coil. If we make

sufficiently large relative to

, then presumably energy storage in the fringing field will be negligible in comparison. Since the alternative leads to a much more complicated problem, we shall assume that Equation 7.13.3 is valid for the interior of the coil.

Next, we determine

. In this case, a natural choice for

is the interior cross-section of the coil in a plane perpendicular to the axis. The direction of

must be

since this is the direction in which the fingers of the right hand point when the current flows in the direction indicated in Figure 7.13.1. Thus, we have

where

is the cross-sectional area of the coil.

Finally from Equation 7.13.1 we obtain

Note that this is dimensionally correct; that is, permeability (units of H/m) times area (units of m²) divided by length (units of m) gives units of H, as expected. Also, it is worth noting that inductance is proportional to permeability and cross-sectional area, and inversely proportional to length. Interestingly the inductance is proportional to

as opposed to

; this is because field strength increases with

, and independently there are

flux linkages. Finally, we note that the inductance does not depend on the shape of the coil cross-section, but only on the area of the cross-section.

Again, this result is approximate because it neglects the non-uniform fringing field near the ends of the coil and the possibility that magnetic field lines escape between windings due to inadequate winding density. Nevertheless, this result facilitates useful engineering analysis and design.

• “Inductance” on Wikipedia.