Electromagnetics I
Ch 9: Plane Waves in Loseless Media
Loc 9.5

Electromagnetics I
Ch 9
Loc 9.5

Uniform Plane Waves - Characteristics

In Section 9.4, expressions for the electric and magnetic fields are determined for a uniform plane wave in lossless media. If the planar phasefront is perpendicular to the

axis, then waves may propagate in either the

direction of the

direction. If we consider only the former, and select

to point in the

direction, then we find

where

is the phase propagation constant,

is the wave impedance, and

is a complex-valued constant associated with the magnitude and phase of the source. This result is in fact completely general for uniform plane waves, since any other possibility may be obtained by simply rotating coordinates. In fact, this is pretty easy because (as determined in Section 9.4)

, and the direction of propagation are mutually perpendicular, with the direction of propagation pointing in the same direction as

In this section, we identify some important characteristics of uniform plane waves, including wavelength and phase velocity. Chances are that much of what appears here will be familiar to the reader; if not, a quick review of Sections 1.3 (“Fundamentals of Waves”) and 3.8 (“Wave Propagation on a Transmission Line”) are recommended.

First, recall that

and

are phasors representing physical (real-valued) fields and are not the field values themselves. The actual, physical electric field intensity is

where

is the phase of

. Similarly:

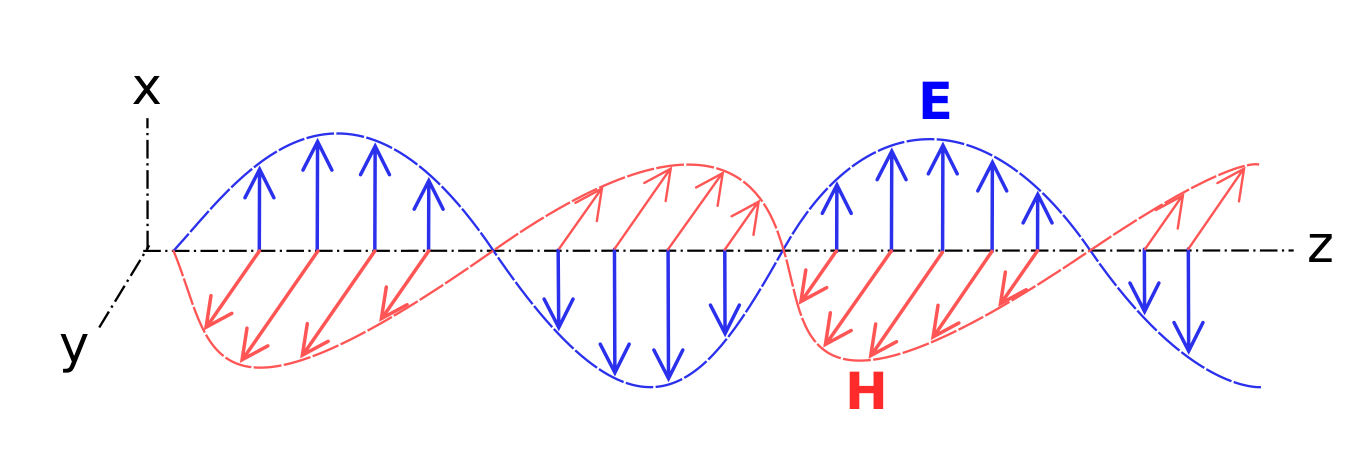
Figure 9.5.1: Relationship between the electric field direction, magnetic field direction, and direction of propagation (here,

). Image used with permission (CC BY SA 3.0; E. Boutet).

This result is illustrated in Figure 9.5.1. Note that both

and

(as well as their phasor representations) have the same phase and have the same frequency and position dependence

. Since

is a real-valued constant for lossless media, only the phase, and not the magnitude, varies with

. If

, then

and the fields no longer depend on

; in this case, the field is not propagating. For

is periodic in

; specifically, it has the same value each time

increases or decreases by

. This is, by definition, the wavelength

If we observe this wave at some fixed point (i.e., hold

constant), we find that the electric and magnetic fields are also periodic in time; specifically, they have the same value each time

increases by

. We may characterize the speed at which the wave travels by comparing the distance required to experience

of phase rotation at a fixed time, which is

; to the time it takes to experience

of phase rotation at a fixed location, which is

. This is known as the phase velocity¹

Note that

has the units expected from its definition, namely (rad/s)/(rad/m) == m/s. If we make the substitution

, we find

Note that

, like the wave impedance

, depends only on material properties. For example, the phase velocity of an electromagnetic wave in free space, given the special symbol

, is

This constant is commonly referred to as the speed of light, but in fact it is the phase velocity of an electromagnetic field at any frequency (not just optical frequencies) in free space. Since the permittivity

and permeability

of any material is greater than that of a vacuum,

in any material is less than the phase velocity in free space.

Summarizing:

Phase velocity is the speed at which any point of constant phase appears to travel along the direction of propagation.

Phase velocity is maximum (

) in free space, and slower by a factor of

in any other lossless medium.

Finally, we note the following relationship between frequency

, wavelength, and phase velocity:

Thus, given any two of the parameters f,

, and

, we may solve for the remaining quantity. Also note that as a consequence of the inverse-proportional relationship between

and

, we find:

At a given frequency, the wavelength in any material is shorter than the wavelength in free space.

Exercise

EXAMPLE 9.5.1: WAVE PROPAGATION IN A LOSSLESS DIELECTRIC.

Polyethylene is a low-loss dielectric having

. What is the phase velocity in polyethylene? What is wavelength in polyethylene? The frequency of interest is 1 GHz.

Low-loss dielectrics exhibit

and

. Therefore the phase velocity is

i.e., very nearly two-thirds of the speed of light in free space. The wavelength at 1 GHz is

Again, about two-thirds of a wavelength at the same frequency in free space.

Returning to polarization and magnitude, it is useful to note that Equation 9.5.3 could be written in terms of E as follows:

i.e.,

is perpendicular to both the direction of propagation (here,

) and

, with magnitude that is different from

by the wave impedance η. This simple expression is very useful for quickly determining

given

when the direction of propagation is known. In fact, it is so useful that it commonly explicitly generalized as follows:

where

(here,

) is the direction of propagation. Similarly, given

, and

, we may find

using

These spatial relationships can be readily verified using Figure 9.5.1.

Equations 9.5.12 and 9.5.13 are known as the plane wave relationships.

The plane wave relationships apply equally well to the phasor representations of

and

; i.e.,

These equations can be readily verified by applying the definition of the associated phasors (e.g., Equation 9.78). It also turns out that these relationships apply at each point in space, even if the waves are not planar, uniform, or in lossless media.

Figure 9.5.2: : A radially-directed plane wave.

Exercise

EXAMPLE 9.5.2: ANALYSIS OF A RADIALLY-DIRECTED PLANE WAVE.

Consider the scenario illustrated in Figure 9.5.2. Here a uniform plane wave having frequency

GHz is propagating along a path of constant

, where is

is known but not specified. The phase of the electric field is

radians at

(the origin) and

. The material is an effectively unbounded region of free space. The electric field is oriented in the

direction and has peak magnitude of 1 mV/m. Find (a) the electric field intensity in phasor representation, (b) the magnetic field intensity in phasor representation, and (c) the actual, physical electric field along the radial path.

First, realize that this “radially-directed” plane wave is in fact a plane wave, and not a cylindrical wave. It may well we be that if we “zoom out” far enough, we are able to perceive a cylindrical wave (for more on this idea, see Section 9.3), or it might simply be that wave is exactly planar, and cylindrical coordinates just happen to be a convenient coordinate system for this application. In either case, the direction of propagation

and the solution to this example will be the same.

Here’s the phasor representation for the electric field intensity of a uniform plane wave in a lossless medium, analogous to Equation 9.5.1:

From the problem statement,

mV/m. Also from the problem statement, the phase of

radians; in fact, we could just write

. Thus, the answer to (a) is

where the propagation constant

rad/m.

The answer to (b) is easiest to obtain from the plane wave relationship:

where

here is

. Thus, the answer to part (b) is

where

A/m. At this point you should check vector directions:

should point in the direction of propagation

. Here we find

, as expected.

The answer to (c) is obtained by applying the defining relationship for phasors to the answer to part (a):

Footnotes

1
We acknowledge that this is a misnomer, since velocity is properly defined as speed in a specified direction, and vp by itself does not specify direction. In fact, the phase velocity in this case is properly said to be +zˆvp. Nevertheless, we adopt the prevailing terminology.