Electric Potential Energy
LEARNING OBJECTIVES
By the end of this section, you will be able to:
- Define the work done by an electric force
- Define electric potential energy
- Apply work and potential energy in systems with electric charges
When a free positive charge q is accelerated by an electric field, it is given kinetic energy (Figure 3.1.1). The process is analogous to an object being accelerated by a gravitational field, as if the charge were going down an electrical hill where its electric potential energy is converted into kinetic energy, although of course the sources of the forces are very different. Let us explore the work done on a charge q by the electric field in this process, so that we may develop a definition of electric potential energy.
(Figure 3.1.1)
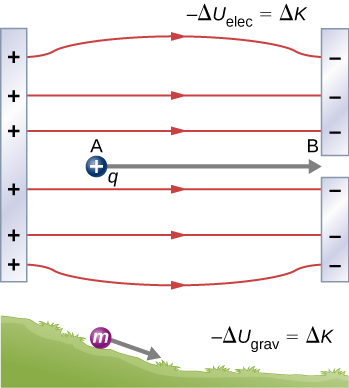


The electrostatic or Coulomb force is conservative, which means that the work done on

is independent of the path taken, as we will demonstrate later. This is exactly analogous to the gravitational force. When a force is conservative, it is possible to define a potential energy associated with the force. It is usually easier to work with the potential energy (because it depends only on position) than to calculate the work directly.
To show this explicitly, consider an electric charge

fixed at the origin and move another charge

toward

in such a manner that, at each instant, the applied force

exactly balances the electric force

on

(Figure 3.1.2). The work done by the applied force

on the charge

changes the potential energy of

. We call this potential energy the electrical potential energy of

.
(Figure 3.1.2)
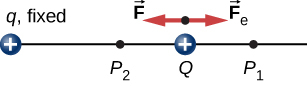


The work

done by the applied force

when the particle moves from

to

may be calculated by

Since the applied force

balances the electric force

on

, the two forces have equal magnitude and opposite directions. Therefore, the applied force is

where we have defined positive to be pointing away from the origin and

is the distance from the origin. The directions of both the displacement and the applied force in the system in Figure 3.1.2 are parallel, and thus the work done on the system is positive.
We use the letter

to denote electric potential energy, which has units of joules (

). When a conservative force does negative work, the system gains potential energy. When a conservative force does positive work, the system loses potential energy,

. In the system in Figure 3.1.2, the Coulomb force acts in the opposite direction to the displacement; therefore, the work is negative. However, we have increased the potential energy in the two-charge system.
EXAMPLE 3.1.1
Kinetic Energy of a Charged Particle
A

charge

is initially at rest a distance of

(

) from a

charge

fixed at the origin (Figure 3.1.3). Naturally, the Coulomb force accelerates

away from

, eventually reaching

(

).
(Figure 3.1.3)



- What is the work done by the electric field between
and
?
- How much kinetic energy does
have at
?
Strategy
Calculate the work with the usual definition. Since

started from rest, this is the same as the kinetic energy.
Solution
Integrating force over distance, we obtain

This is also the value of the kinetic energy at

.
Significance
Charge

was initially at rest; the electric field of

did work on

, so now

has kinetic energy equal to the work done by the electric field.
CHECK YOUR UNDERSTANDING 3.1
If
has a mass of
what is the speed of
at
?In this example, the work
done to accelerate a positive charge from rest is positive and results from a loss in
, or a negative
. A value for
can be found at any point by taking one point as a reference and calculating the work needed to move a charge to the other point.
ELECTRIC POTENTIAL ENERGY
Work

done to accelerate a positive charge from rest is positive and results from a loss in

, or a negative

. Mathematically,

(3.1.1)
Gravitational potential energy and electric potential energy are quite analogous. Potential energy accounts for work done by a conservative force and gives added insight regarding energy and energy transformation without the necessity of dealing with the force directly. It is much more common, for example, to use the concept of electric potential energy than to deal with the Coulomb force directly in real-world applications.
In polar coordinates with

at the origin and

located at

, the displacement element vector is

and thus the work becomes

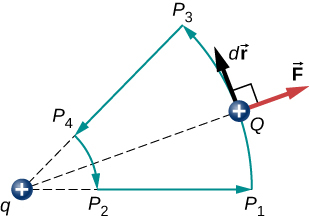
Notice that this result only depends on the endpoints and is otherwise independent of the path taken. To explore this further, compare path

to

with path

in Figure 3.1.4.
(Figure 3.1.4)







The segments

and

are arcs of circles centred at

. Since the force on

points either toward or away from

, no work is done by a force balancing the electric force, because it is perpendicular to the displacement along these arcs. Therefore, the only work done is along segment

, which is identical to

One implication of this work calculation is that if we were to go around the path

, the net work would be zero (Figure 3.1.5). Recall that this is how we determine whether a force is conservative or not. Hence, because the electric force is related to the electric field by

, the electric field is itself conservative. That is,

Note that

is a constant.
(Figure 3.1.5)
Another implication is that we may define an electric potential energy. Recall that the work done by a conservative force is also expressed as the difference in the potential energy corresponding to that force. Therefore, the work

to bring a charge from a reference point to a point of interest may be written as

and, by Equation 3.1.1, the difference in potential energy (

) of the test charge

between the two points is

Therefore, we can write a general expression for the potential energy of two point charges (in spherical coordinates):

We may take the second term to be an arbitrary constant reference level, which serves as the zero reference:

A convenient choice of reference that relies on our common sense is that when the two charges are infinitely far apart, there is no interaction between them. Taking the potential energy of this state to be zero removes the term

from the equation (just like when we say the ground is zero potential energy in a gravitational potential energy problem), and the potential energy of

when it is separated from

by a distance

assumes the form

(3.1.2)
This formula is symmetrical with respect to

and

, so it is best described as the potential energy of the two-charge system.
EXAMPLE 3.1.2
Potential Energy of a Charged Particle
A

charge

is initially at rest a distance of

(

) from a

charge

fixed at the origin (??). Naturally, the Coulomb force accelerates

away from

, eventually reaching

(

).
(Figure 3.1.6)
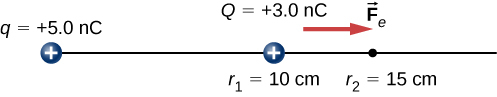


What is the change in the potential energy of the two-charge system from

to

?
Strategy
Calculate the potential energy with the definition given above:

. Since

started from rest, this is the same as the kinetic energy.
Solution
We have

Significance
The change in the potential energy is negative, as expected, and equal in magnitude to the change in kinetic energy in this system. Recall from Example 3.1.1 that the change in kinetic energy was positive.
CHECK YOUR UNDERSTANDING 3.2
What is the potential energy of

relative to the zero reference at infinity at

in the above example?
Due to Coulomb’s law, the forces due to multiple charges on a test charge

superimpose; they may be calculated individually and then added. This implies that the work integrals and hence the resulting potential energies exhibit the same behaviour. To demonstrate this, we consider an example of assembling a system of four charges.
EXAMPLE 3.1.3
Assembling Four Positive Charges
Find the amount of work an external agent must do in assembling four charges

,

,

, and

at the vertices of a square of side

, starting each charge from infinity (Figure 3.1.7).
(Figure 3.1.7)
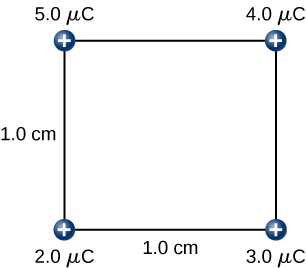
Strategy
We bring in the charges one at a time, giving them starting locations at infinity and calculating the work to bring them in from infinity to their final location. We do this in order of increasing charge.
Solution
Step 1. First bring the

charge to the origin. Since there are no other charges at a finite distance from this charge yet, no work is done in bringing it from infinity,

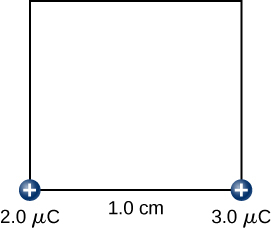
Step 2. While keeping the

charge fixed at the origin, bring the

charge to

(Figure 3.1.8). Now, the applied force must do work against the force exerted by the

charge fixed at the origin. The work done equals the change in the potential energy of the

charge:

(Figure 3.1.8)


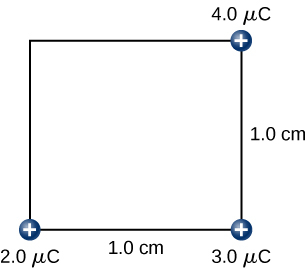
Step 3. While keeping the charges of

and

fixed in their places, bring in the

charge to

(Figure 3.1.9). The work done in this step is

Figure 3.1.9
Step 4. Finally, while keeping the first three charges in their places, bring the

charge to

(Figure 3.1.10). The work done here is

(Figure 3.1.10)
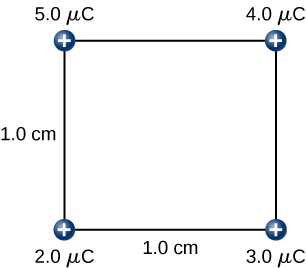
Hence, the total work done by the applied force in assembling the four charges is equal to the sum of the work in bringing each charge from infinity to its final position:

Significance
The work on each charge depends only on its pairwise interactions with the other charges. No more complicated interactions need to be considered; the work on the third charge only depends on its interaction with the first and second charges, the interaction between the first and second charge does not affect the third.
CHECK YOUR UNDERSTANDING 3.3
Is the electrical potential energy of two point charges positive or negative if the charges are of the same sign? Opposite signs? How does this relate to the work necessary to bring the charges into proximity from infinity?
Note that the electrical potential energy is positive if the two charges are of the same type, either positive or negative, and negative if the two charges are of opposite types. This makes sense if you think of the change in the potential energy

as you bring the two charges closer or move them farther apart. Depending on the relative types of charges, you may have to work on the system or the system would do work on you, that is, your work is either positive or negative. If you have to do positive work on the system (actually push the charges closer), then the energy of the system should increase. If you bring two positive charges or two negative charges closer, you have to do positive work on the system, which raises their potential energy. Since potential energy is proportional to

, the potential energy goes up when

goes down between two positive or two negative charges.
On the other hand, if you bring a positive and a negative charge nearer, you have to do negative work on the system (the charges are pulling you), which means that you take energy away from the system. This reduces the potential energy. Since potential energy is negative in the case of a positive and a negative charge pair, the increase in

makes the potential energy more negative, which is the same as a reduction in potential energy.
The result from Example 3.1.1 may be extended to systems with any arbitrary number of charges. In this case, it is most convenient to write the formula as

(3.1.3)
The factor of 1/2 accounts for adding each pair of charges twice.
Candela Citations
CC licensed content, Specific attribution
- Download for free at http://cnx.org/contents/7a0f97...; Retrieved from: http://cnx.org/contents/7a0f97...; License: CC BY: Attribution
Introduction to Electricity, Magnetism, and Circuits by Daryl Janzen is licensed under a Creative Commons Attribution 4.0 International License, except where otherwise noted.
Explore CircuitBread

Get the latest tools and tutorials, fresh from the toaster.







