- Electromagnetics I
- Ch 3
- Loc 3.10
Coaxial Line
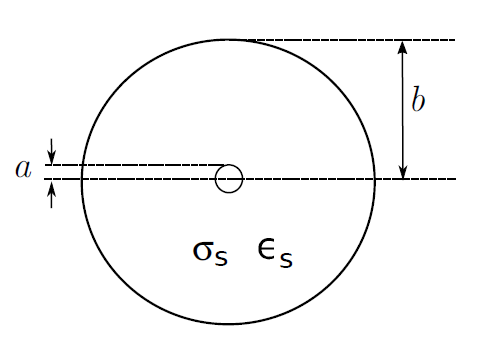
Coaxial transmission lines consists of metallic inner and outer conductors separated by a spacer material as shown in Figure 3.10.1
. The spacer material is typically a low-loss dielectric material having permeability approximately equal to that of free space (

) and permittivity

that may range from very near

(e.g., air-filled line) to 2–3 times

. The outer conductor is alternatively referred to as the “shield,” since it typically provides a high degree of isolation from nearby objects and electromagnetic fields. Coaxial line is single-ended1 in the sense that the conductor geometry is asymmetric and the shield is normally attached to ground at both ends. These characteristics make coaxial line attractive for connecting single-ended circuits in widely-separated locations and for connecting antennas to receivers and transmitters.
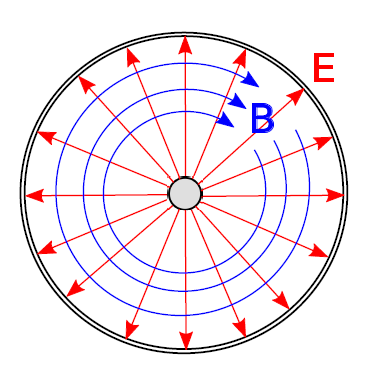
Coaxial lines exhibit TEM field structure as shown in Figure 3.10.2.
Expressions for the equivalent circuit parameters

and

for coaxial lines can be obtained from basic electromagnetic theory. It is shown in Section 7.14 that the capacitance per unit length is

where

and

are the radii of the inner and outer conductors, respectively. Using analysis shown in Section 7.14, the inductance per unit length is

The loss conductance

depends on the conductance σsσs of the spacer material, and is given by

This expression is derived in Section 6.5.
The resistance per unit length,

, is relatively difficult to quantify. One obstacle is that the inner and outer conductors typically consist of different materials or compositions of materials. The inner conductor is not necessarily a single homogeneous material; instead, the inner conductor may consist of a variety of materials selected by trading-off between conductivity, strength, weight, and cost. Similarly, the outer conductor is not necessarily homogeneous; for a variety of reasons, the outer conductor may instead be a metal mesh, a braid, or a composite of materials. Another complicating factor is that the resistance of the conductor varies significantly with frequency, whereas

,

, and

exhibit relatively little variation from their electro- and magnetostatic values. These factors make it difficult to devise a single expression for

that is both as simple as those shown above for the other parameters and generally applicable. Fortunately, it turns out that the low-loss conditions

and

are often applicable2, so that

and

are important only if it is necessary to compute loss.
Since the low-loss conditions are often met, a convenient expression for the characteristic impedance is obtained from Equations 3.10.1 and 3.10.2 for

and

respectively:

The spacer permittivity can be expressed as

where

is the relative permittivity of the spacer material. Since

is a constant, the above expression is commonly written

Thus, it is possible to express

directly in terms of parameters describing the geometry (

and

) and material (

) used in the line, without the need to first compute the values of components in the lumped-element equivalent circuit model.
Similarly, the low-loss approximation makes it possible to express the phase velocity

directly in terms the spacer permittivity:

since

. In other words, the phase velocity in a low-loss coaxial line is approximately equal to the speed of electromagnetic propagation in free space, divided by the square root of the relative permittivity of the spacer material. Therefore, the phase velocity in an air-filled coaxial line is approximately equal to speed of propagation in free space, but is reduced in a coaxial line using a dielectric spacer.
Exercise
RG-59 Coaxial Cable
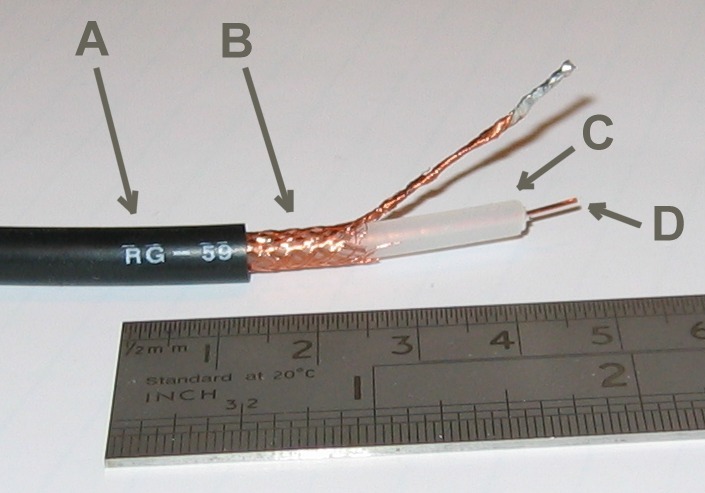
RG-59 is a very common type of coaxial line. Figure 3.10.3 shows a section of RG-59 cut away so as to reveal its structure. The radii are

mm and

mm (mean), yielding

nH/m. The spacer material is polyethylene having

, yielding

pF/m. The conductivity of polyethylene is

S/m, yielding

S/m. Typical resistance per unit length

is on the order of

/m near DC, increasing approximately in proportion to the square root of frequency.
From the above values, we find that RG-59 satisfies the low-loss criteria

for

kHz and

for

kHz. Under these conditions, we find

. Thus, the ratio of the potential to the current in a wave traveling in a single direction on RG-59 is about

.
The phase velocity of RG-59 is found to be

m/s, which is about 67% of

. In other words, a signal that takes 1 ns to traverse a distance

in free space requires about 1.5 ns to traverse a length-

section of RG-59. Since

, a wavelength in RG-59 is 67% of a wavelength in free space.
Using the expression

with

/m, and then taking the real part to obtain

, we

m−1. So, for example, the magnitude of the potential or current is decreased by about 50% by traveling a distance of about 70 m. In other words,

for

m at relatively low frequencies, and increases with increasing frequency.
Footnotes
Additional Reading
- “Coaxial cable” on Wikipedia. Includes descriptions and design parameters for a variety of commonly-encountered coaxial cables.
- “Single-ended signaling” on Wikipedia.
- Sec. 8.7 (“Differential Circuits”) in S.W. Ellingson, Radio Systems Engineering, Cambridge Univ. Press, 2016.
Ellingson, Steven W. (2018) Electromagnetics, Vol. 1. Blacksburg, VA: VT Publishing. https://doi.org/10.21061/electromagnetics-vol-1 CC BY-SA 4.0
Explore CircuitBread

Get the latest tools and tutorials, fresh from the toaster.







